I was thrilled that so many showed up! For those who were unable to make it (and for those who did and would like the files), here’s a run-through of what my session was about. Sorry about the length of this post – I’m trying to re-live the workshop! Here’s an overall outline in case you wish to jump to a particular section:
- Principles of Task Design
- Pattern Exploration
- Divisibility by 9
- Big Idea about Operations
- Multiplication
- Division
- Division of Fractions
- Simplifying Radicals
First a few files.
- Here’s the handout that I provided for participants to play along for some of the activities.
- If you have Keynote, here are my slides. Note that this is for Keynote ’09. The newer version misses some of the table cell fills.
- Or here is a pdf version of the slides, sans animations of course.
I was honoured to have my session chosen for the Learn↔Reflect strand. Here are the questions that were guiding the strand (with emphasis added):
- What is number sense, and how can you promote the development of number sense in your students? How are fluency and understanding related in the context of number and operations?
- How can instructional decisions facilitate the development of strategies that are meaningful and transferable for operations on all numbers?
- How are equity and diversity promoted by developing conceptual understanding of number?
- How can the Standards for Mathematical Practice support the development of number sense and computational fluency?
- How are you thinking differently about your learning and teaching of number and operations as a result of participating in the Learn↔Reflect sessions?
My Principles of Task Design
I had some messages that I wanted to thread throughout my workshop, and I chose the umbrella of “principles” to do so. As such, this is not an exhaustive list.
- Meaningful mathematics
- Context or no – what matters most to me is that there be some real mathematics.
- Sense-making
- This is my goal for my learners – this is what I think defines success, much more than getting right answers. Does the math make sense? And how? And what next?
- Who’s doing the math?
- For years my reflective practice led to trying better and better ways to explain the math. I made everything so clear, identified misconceptions and explained how to avoid them. Ummm… that wasn’t working. It finally hit me, duh!, that I was the one doing the math – my students weren’t doing mathematics, they were just trying to do what I was doing. Sense-making – not!
- “I tell my students…” Oh, how many times I’ve heard that. Not that there aren’t some things which need to be told, but if sense-making is the goal, no amount of telling is going to do it, not for most learners anyways.
- And what does it mean to do math? I like to think of math as a verb. Answer-getting, for its own sake, is not doing math.
Pattern Exploration
I’ve seen so many resources and online lessons which take a pattern-exploration approach to mathematical concepts. This is a step in the right direction. But I believe we can do better. A future post will explore this further, but to give an example, let’s consider divisibility by 9.
Pattern approach:
- Examine numbers divisible by 9 (see slide for examples).
- Add up the digits.
- What do you notice?
Yep, this would hopefully lead to the conclusion of the rule for divisibility by 9. But I think we can do better. And while a nice symbolic proof makes us math people drool – such proofs may cause students to drool in a different way, ie. napping.
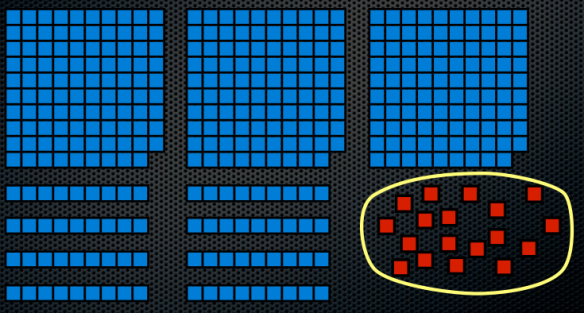
So consider this visual approach to divisibility by 9, with the specific example:
Is the number 387 divisible by 9?
Try making sense of this visually before reading below.
The Keynote slide shows a nice animation of one approach. Here’s the end result:
One way of thinking of dividing by 9 is to make 9 groups. If we take 1 away from each 100, what remains (99) can be divided into 9 groups. Similarly so if we take 1 away from each 10. The overall divisibility by 9 is determined by whether the remaining number of 1s is divisible by 9 (ie. the red ones). And this group is formed by 1 from each hundred, 1 from each ten, and the 1s – uh huh! – it’s the sum of the digits. Extending this to larger numbers is not a difficult generalization to make.
A Big Idea about Number Operations
My colleague Chris Hunter developed an activity that we used for PD for our Secondary Mathematics Department Heads. It was framed around the following big idea:
The operations of addition, subtraction, multiplication, and division hold the same fundamental meaning no matter the domain to which they are applied. (Marian Small)
This is an idea that really came to life as I began to work in elementary classrooms. I encourage all secondary teachers that if you ever get a chance to spend some time thinking about, and even better, teaching elementary math, take it! It will transform your secondary math classroom. I’m so grateful for all I’ve learned from my elementary colleagues!
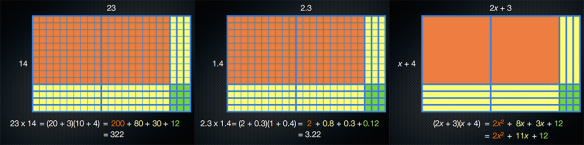
Consider the operation of multiplication, specifically using an area model. The same visualization and sense-making holds whether one be considering whole numbers, decimal numbers, or algebraic binomials (click on the image to view a more legible size):
Now for the next activity in the handout. Evaluate, or simplify, each set of expressions.
Make as many connections as you can:
- conceptually & procedurally
- pictorially & symbolically
Take a moment and think of this before proceeding.
It is helpful when dividing to consider two different meanings of division (consider 6 ÷ 3):
- Partitive: Or sharing – If there are 3 groups, how many are in each group?

- Quotative: Or measurement – If there are groups of 3, how many groups? Or, how many 3s go into 6?

The numerical answers are the same, but the meaning and visualization are different. Consider, which meaning helps make sense of (–6) ÷ (+3) ?
Which meaning makes sense of the fraction question?
Oh how crazy I was when I used to insist to students not to use a common denominator when dividing fractions, only use them for adding or subtracting. A common denominator makes dividing fractions make more sense if we consider the quotative meaning!
I encourage you also to consider common numerator fraction division. Then check out this from Christopher Danielson.
Next a quick example from Marian Small that I leave for you. Think in terms of the meaning of division.
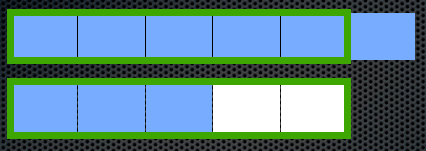
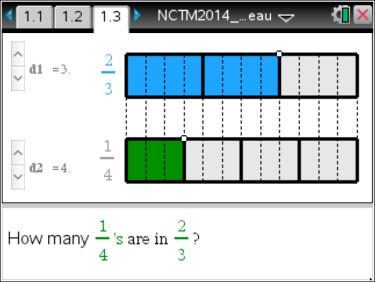
 Next consider this fraction question. A grid was provided if it helped.
Next consider this fraction question. A grid was provided if it helped.
Take some time. How would you represent this division in a visual way?
Here’s one approach:
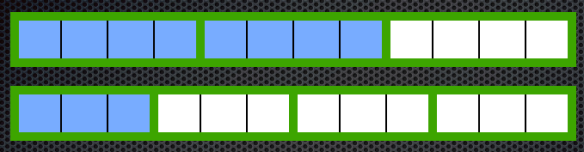 One can make sense of the answers 2 2/3, or 8/3. The key here is the common denominator (not that other approaches can not also make sense). But don’t tell students that that’s what they need – they can be motivated to discover that need. First, they need to have in mind that the wholes for each fraction need to be the same. Then it’s about making sense of how we can reason to how many/much of the divisor goes into the dividend.
One can make sense of the answers 2 2/3, or 8/3. The key here is the common denominator (not that other approaches can not also make sense). But don’t tell students that that’s what they need – they can be motivated to discover that need. First, they need to have in mind that the wholes for each fraction need to be the same. Then it’s about making sense of how we can reason to how many/much of the divisor goes into the dividend.
Here’s a TI-Nspire document that allows one to explore dividing fractions this way. The fractions can be changed using sliders.
I highly encourage you to check out this post from Fawn Nguyen.
A couple of more things here. One, there are other visualizations and representations (e.g.: I think cuisenaire rods and pattern blocks are fantastic) that work well here, and hence I didn’t require that one use the grid. Second, this is the visual piece – I think it’s important to make symbolic connections as well.
Exploring Radicals
I didn’t have as much time as I’d like by this point of the workshop, but given radicals was in the title, we had to get to it! This is also what I feel is the coolest part. See the handout for the lesson. I’ll give a brief summary.
What does square root mean visually?
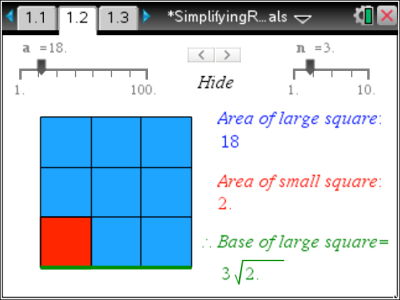
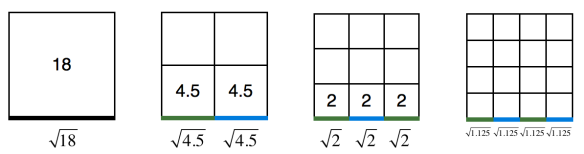
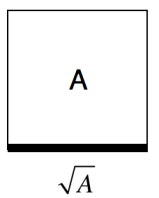 I understood what square root means, but for so many years never thought to make the connection to its geometric meaning, but rather just relied on the numerical meaning when trying to teach about simplifying radicals. Then one night (confession: I was enjoying a beverage with a colleague and what comes next was drawn on a napkin!), it occurred to me that there is a powerful connection between the geometric and numeric meanings of square root. Consider a square whose area is 18. Next consider how we may express the base of that square (ie. the square root) in different ways, visually! The key is to divide the large square into areas that we can express numerically, i.e. into squares, because we can use square roots to denote those lengths.
I understood what square root means, but for so many years never thought to make the connection to its geometric meaning, but rather just relied on the numerical meaning when trying to teach about simplifying radicals. Then one night (confession: I was enjoying a beverage with a colleague and what comes next was drawn on a napkin!), it occurred to me that there is a powerful connection between the geometric and numeric meanings of square root. Consider a square whose area is 18. Next consider how we may express the base of that square (ie. the square root) in different ways, visually! The key is to divide the large square into areas that we can express numerically, i.e. into squares, because we can use square roots to denote those lengths.
All of these bases are the same length. Think about how to express them.
![]()
All of these are true equivalents, but one is the simplest, once we decide on what simplest means. Also note, dividing into squares connects nicely to the numerical strategy of finding a square factor. For more details, check out Chris Hunter’s blog post.
Technology-wise, I’ve created documents for both TI-Nspire and Geogebra. Click on the links to get the files.
 That’s all folks! One other idea I didn’t have time to get to was considering using graphs of log functions to determine logarithmic identities.
That’s all folks! One other idea I didn’t have time to get to was considering using graphs of log functions to determine logarithmic identities.


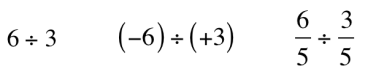
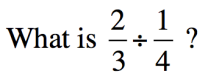
Pingback: Visualizing Mathematics Concepts: A Key to Making Connections | NCTM Meetings